1. Calcusyn Software Free Download

Combination therapies exploit the chances for better efficacy, decreased toxicity, and reduced development of drug resistance and owing to these advantages, have become a standard for the treatment of several diseases and continue to represent a promising approach in indications of unmet medical need. In this context, studying the effects of a combination of drugs in order to provide evidence of a significant superiority compared to the single agents is of particular interest. Research in this field has resulted in a large number of papers and revealed several issues. Here, we propose an overview of the current methodological landscape concerning the study of combination effects.

Calcusyn Software Free Download

First, we aim to provide the minimal set of mathematical and pharmacological concepts necessary to understand the most commonly used approaches, divided into effect-based approaches and dose–effect-based approaches, and introduced in light of their respective practical advantages and limitations. Then, we discuss six main common methodological issues that scientists have to face at each step of the development of new combination therapies. In particular, in the absence of a reference methodology suitable for all biomedical situations, the analysis of drug combinations should benefit from a collective, appropriate, and rigorous application of the concepts and methods reviewed here.

IntroductionTraditional and modern medicine has always taken advantage of the combined use of several active agents to treat different diseases. Based on a practice of more than 2000 years, traditional Chinese medicine uses mixtures of naturally occurring herbs (Yuan ). Since the last century, advances in Omics and Cell Biology have greatly impacted on the increasing use of drug combination in modern medicine (Keith et al. The enhanced understanding of the biology of a disease as a disturbed system of interconnected molecular pathways which are more susceptible to the simultaneous action of several drugs, provides new opportunities for the rational development of combination therapies (Smalley et al.; Kitano; Zimmermann et al.; Podolsky and Greene ) and exploits the chances for better efficacy, decreased toxicity, and reduced development of drug resistance.For these reasons, combination therapies have become a standard in several areas such as cancer (Humphrey et al. ), hypertension (Glass ), asthma (Nelson ), and AIDS (Larder et al.; Oversteegen et al. In addition, for the pharmaceutical industry which is currently facing a decline in the discovery and approval of new molecular entities, reformulating existing drugs into combination products represents an essential strategy in indications of unmet medical need such as Alzheimer’s disease (Herrick and Million; Pangalos et al. ).For this purpose providing evidence of significant superiority of a combination of drugs compared to the single agents is of particular interest.

Calcusyn 2.1 serial numbers, cracks and keygens are presented here. No registration is needed. Just download and enjoy. It is based on the same Chou-Talalay’s Combination Index Theorem that CalcuSyn is based on. It is for PC and requires Java. There is a brief registration, and then its all yours!! CalcuSyn Version 2.0 is the definitive analyzer of combined drug effects, able automatically to quantify phenomena such as synergism and inhibition. Mixed drug treatment is becoming common in the treatment of cancer, AIDS etc, and CalcuSyn is the most widely used.

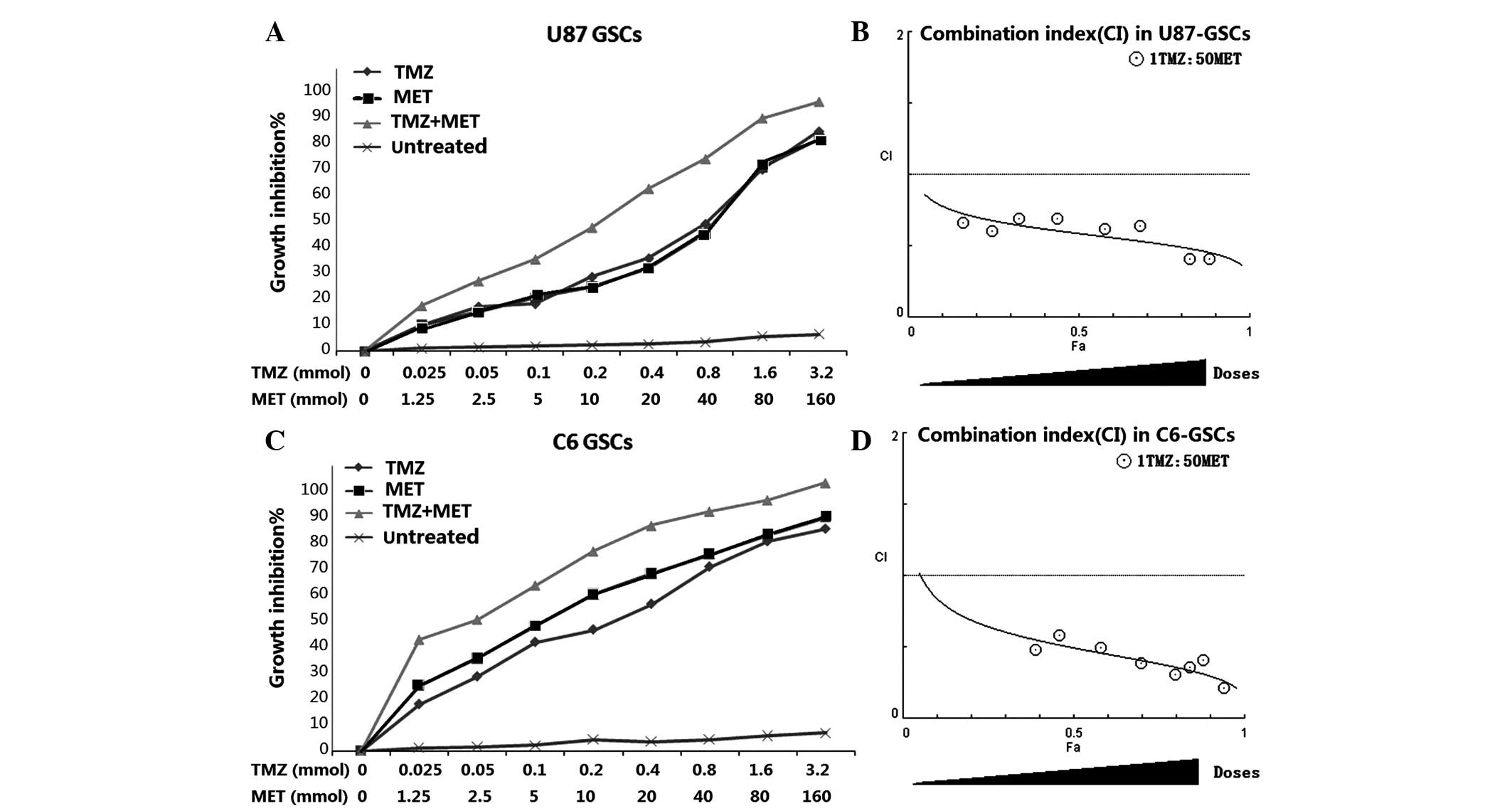
Research in this field has resulted in a large number of theoretical and experimental papers (Greco et al.; Chou ), and also revealed several methodological issues and caveats (Berenbaum; Caudle and Williams; Nieuwenhuis et al.; Ocana et al.; Geary ). In particular the concepts of synergy or antagonism have clear and well-accepted definitions: they represent, respectively, greater or lesser effects for drugs in combination than the simple additive effect expected from the knowledge of the effects of each drug individually. Effect-Based StrategyMethods following an effect-based strategy compare the effect resulting from the combination of two drugs ( E AB) directly to the effects of its individual components ( E A and E B). The exact decision process that allows a conclusion of positive, negative, or null combination effect can vary among four main strategies which are (1) Combination Subthresholding, (2) Highest Single Agent, (3) Response Additivity, and (4) Bliss Independence model described hereafter and illustrated in Figure. Possible inconsistency in assessing drug synergy based on Response Additivity or Bliss Independence. Identical simulated dose–effect curve for two different drugs.

Suppose that a dose = 4 of drug A results in 25% of effect, likewise for drug B. From Response Additivity, one would conclude in synergism with a combination effect above 50%. From Bliss Independence, one would conclude in synergism with a combination effect above 43%. However, note that either a dose = 2 × 4 = 8 of drug A or of drug B alone brings the effect up to 91%. Therefore, a total of dose = 8 of the hypothetical combined drug elicits less effect under Response Additivity or Bliss Independence than the same dose of either drug alone, yet one would conclude synergism.The Bliss Independence model (Bliss; Berenbaum; Greco et al.; Geary ) is based on the principle that drug effects are outcomes of probabilistic processes and assumes that drugs act independently in such a manner that neither of them interferes with the other (different sites of action), but each contributes to a common result (Fig.).

The observed combination effect expressed as a probability (0 ≤ E AB ≤ 1) can be compared to the expected additive effect given by the common formula for probabilistic independence E A + E B(1 − E A) = E A + E B − E A E B, where 0 ≤ E A ≤ 1 and 0 ≤ E B ≤ 1. The resulting Combination Index can be calculated as:. The Bliss Independence model is considered as one of the most popular models to assess the combined effects of drugs, but it presents some limitations (Goldoni and Johansson ). First, the search for synergy often involves drugs with multiple, complex, possibly unknown mechanisms of action, and therefore, methodologies should not depend upon knowledge of mechanisms of action (Greco et al. Then, Bliss Independence assumes that the drugs have exponential dose–effect curves (Berenbaum ), which could lead the same counterintuitive interpretation discussed for Response Additivity and illustrated in Figure. Finally a main limitation is that the model applies only to effects expressed as probabilities ranging within 0 and 1. Dose–Effect-Based StrategyOpponents to the effect-based approaches consider that the proper way to compare different agents having nonlinear dose–effect curves is to find what amount or concentration of each produces the same quantitative effect, which can be referred to as dose–effect-based approaches (Berenbaum ).

The expected (additive) effect of a combination depends on the individual dose–effect curves and enables the formulation of unequivocal definitions of synergy, additivism, and antagonism. In particular dose–effect-based approaches rely on the mathematical framework known as Loewe Additivity, since it was first mentioned by Frei (Frei ) but first defined formally by Loewe (Loewe, ).

Where E A is measured on the dose–effect curve of drug A, ( a + a b) corresponds to the dose A giving the effect E AB and, respectively, for drug B. It makes the assumption that the drugs have a constant potency ratio ( ).

In practice, dose–effect curves with constant potency ratio have a constant ratio of doses at every level of effect and hence are parallel on a log-dose scale, and have equal individual drug maximum effects (Fig.) (Tallarida ). From there, we can easily define the following relation between all pairs of doses ( a, b) producing the combination effect E AB and the single doses A and B necessary to reach this effect. Illustration of the Loewe Additivity. (A) Dose–effect curves for two drugs A and B (here with constant potency ratio R) allow estimation of the single doses A E and B E reaching the combination effect E produced by the combination of doses a of drug A and b of drug B. (B) Isobologram analysis at the combination effect E.

The single doses A E and B E are used to draw the line of additivity. The localization of the experimental point ( a, b) corresponding to the doses actually needed for a combination effect E with respect to the line of additivity can be translated in term of synergy, additivity, and antagonism. Combination indexThis relation first allows us to assess a Combination Index for the Loewe Additivity (Berenbaum; Chou and Talalay, ):. In practice a CI  1 indicates that the doses a and b producing a given effect in combination are superior to the expected doses from additivity and can hence be directly interpreted as antagonism. Isobologram analysisAnother advantage of the Loewe Additivity is that it also enables us to complement the algebraic analysis with an intuitive, flexible and widely accepted graphical approach known as isobologram analysis (Greco et al.

Given an effect E produced by the combination of doses a of drug A and b of drug B, the equation defines all the pairs of doses of drugs A and B that should lead to the combination effect E AB from additivity, and can be drawn as a line of additivity of negative slope (, also called additive isobole) on a graph where the x and y axes represent the dose of drugs A and B (Fig.). This representation makes clear that when drug A is present at dose A the quantity of drug B needed to reach the specified level is zero, and that the presence of drug B reduces the need for drug A in a quantity predicted by the model.

Then the localization of the experimental point ( a, b) corresponding to the doses actually needed for a combination effect E AB with respect to the line of additivity can be translated in term of synergy, additivity and antagonism (Grabovsky and Tallarida; Chou; Geary ): an experimental point below the line corresponds to a CI  1 and indicates antagonism (Fig.). Issue 1: the analysis of drug combinations requires an appropriate use of concepts and methodsThe term “synergy” is used extensively as a gold standard to justify drug combinations when designing clinical studies. However, it has been shown that the literature is often obscure and is profusely littered with technical terms that are not always clearly defined (Berenbaum ), and that in most studies, the term “synergy” is used without appropriate understanding of either the underlying concept or the methods necessary to evaluate it (Ocana et al.; Berthoud ). It is clear from the paradoxes illustrated in Figure that the interpretation of drug combination effects requires a minimal knowledge of the single dose–effect curves within the range of effect of interest (Berenbaum; Greco et al.; Tallarida; Goldoni and Johansson; Lee and Kong; Ocana et al. Experiments are commonly designed in such a way that they could not detect synergy even if it was present and results are interpreted as showing synergy when there is no evidence for it or as showing additivity where there is clear antagonism, and so on (Berenbaum ). Experiments in which any drug is tested at less than three dose levels are therefore not likely to be sufficient to demonstrate synergy (Berenbaum ).

It is also worthy of mention that the positive combination effect of two drugs can take other forms than synergy. When only one drug is active alone, a greater combination effect is generally referred to as “ potentiation.” When the drugs combined are not active alone an effective combination is termed “ coalism.” A combination can also have an effect on a range of biological systems or anatomical sites that are not completely covered by any drug individually, a situation described as “ cooperation” (Gordon Steel and Peckham ). Issue 2: the analysis of drug combinations requires a standard reference analysis frameworkThis framework should ideally (a) provide a clear definition of additivity, synergy, and antagonism, (b) not rely on knowledge of mechanisms of action that are often unknown or not well understood even for many common drugs such as aspirin (Jia et al.

), (c) be general enough to cover rare and specific cases, (d) not result in counterintuitive results, (e) be adapted to possible practical and ethical issues to obtain data, (f) be intuitive and user friendly to be adopted by most scientists. There is to date no universal method that fulfills all the aspects of this task and although useful, “all models are wrong” (Box and Draper; Shafer ). Dose–effect approaches based on Loewe Additivity can best survive criticism (Greco et al. ), but the relatively large amount of data required can make combination experiments prohibitive when data are expensive or difficult to obtain. Effect-based approaches such as Highest Single Agent, Response Additivity, and Bliss Independence appear more adapted to practical limitations as one minimally needs three or four experimental points for their application: (0, 0), ( a, 0), (0, b), and ( a, b).

Despite their limitations, they can provide sufficient and convincing evidence of positive combination effect. In this context, we feel that the search for a reference analysis framework will not find its solution in one ideal model but rather in using a set of appropriate methods adapted to each step of the research and development process from discovery to the marketing authorization application, as discussed in the next issue. Issue 4: optimizing dose ratioThe benefit of a combination therapy is not simply due to the property of the drugs, but could also depend on the dose ratio. As the cells do not make the difference between a single drug or a combination, two drugs combined at a given ratio could be considered as a third agent with its own dose–effect relation (Chou ). Therefore, rather than simply asking whether a particular combination is synergistic, we might do better to consider what dose ratio optimizes the synergy (Keith et al. For this purpose, a multiple-ray design (Fig.) exploring a given set of fixed ratios (the dose of one drug is escalated while the dose of the second remains constant) should be preferred to the full factorial design (Fig.) considering all the combinations of the selected doses of the individual drugs (Chou and Talalay; Greco et al.; Straetemans et al.

From there different dose ratios can be compared by the mean of their respective dose–effect curves by applying a curve-shift analysis (Fig.) (Zhao et al. ), and a 3D response-surface analysis spanning the explored region of doses can provide a more complete description of the combination effect (Fig.) (Prichard and Shipman; Greco et al.; Breitinger; Geary ). Ideally, the dose ratio should be optimized in preclinical studies before proceeding to clinical testing in humans.

Optimizing dose ratio. (A) Multiple-ray design exploring 16 combinations (4 ratios × 4 doses). (B) Full factorial design exploring 16 combinations (4 × 4 doses). (C) Curve-shift analysis. The dose–effect curve for a combination at a given ratio (in purple) is compared to the additive expectation (in red) which can illustrate synergy by both an increase in potency and/or an increase in efficacy relatively to the single agent responses. Additive and combination curves are represented as functions of the dose of the more potent drug (here drug A).

(D) Response-surface analysis can provide a complete description of the combination effect over a large range of doses. Issue 6: combining more than two drugsDrug combination analysis is often presented on drug pairs in order to ease its understanding and because it covers the most common situation in practice. But combining more than two drugs is not so rare (in cancers chemotherapy regiments can easily reach four or five agents) and most of the methods described here can easily be extended for use with any number of drugs (Bliss; Berenbaum; Chou and Talalay ). For instance with more than two drugs combined, the Combination Index is generalized to:.for the Highest Single Agent approach,.for the Bliss Independence model,.for the Loewe Additivity.

Note that when the combination counts three drugs, the equation CI = 1 corresponds to the plane passing through A, B, and C when doses of drugs A, B, and C are, respectively, presented by three coordinate axes, instead of a line when combining two drugs.However, such a generalization to the analysis of more than two drugs does not allow investigation of the contribution of each drug to the whole combination effect. A combination of three drugs (A, B, and C) with a synergistic effect (CI. Conclusions and ProspectsDrug combination effects have been studied and analyzed by scientists for over 100 years. The advantages of combining drugs are well recognized, and activity in the area has increased dramatically thanks to the opportunities provided by the enhanced understanding of Systems Biology of disease (Keith et al.; Zimmermann et al. This research has resulted in an immense number of theoretical and experimental papers in nearly all biological and medical sciences, and has involved scientists from many disciplines (Pharmacology, Mathematics, Epidemiology, and others) (Greco et al.

Methods to generate and analyze data have evolved substantially over time thanks to substantial improvements in screening technologies and computational capacities (Cokol et al. ), but the main methodological issues remain appreciably the same (Prichard and Shipman ). As a significant part of the theoretical and empirical literature propagates fundamental misunderstandings (Berenbaum,; Caudle and Williams; Nieuwenhuis et al.; Ocana et al.; Berthoud; Geary ), future developments should benefit from a more appropriate and rigorous application of the concepts and methods discussed here for a proper assessment and interpretation of combination effects. In addition, in the absence of a reference methodology appropriate for all biomedical situations, the analysis of drug combinations will be facilitated by the collective use of different approaches. Finally, and beside the study design and analysis methodological aspects discussed here, research and pharmaceutical companies working on new combination therapies will have to face additional challenges such as elucidating the mechanisms of action by which drugs cause their single and joint actions (Jia et al. ) and improving the science behind formulating combination drugs.

Thank you for downloading CalcuSyn from our software libraryThe version of CalcuSyn you are about to download is 2.0. The contents of the download are original and were not modified in any way. The software is licensed as trial. Please bear in mind that the use of the software might be restricted in terms of time or functionality. The download was scanned for viruses by our system.

We also recommend you to check the files before installation. CalcuSyn antivirus reportThis download is virus-free.This file was last analysed by Free Download Manager Lib 68 days ago.